I received an email in late August 2019 from my former boss at KTH, Professor Peter Händel. He had been working for many years on the modeling of hardware imperfections in wireless transceivers. Our research journeys had recently crossed since he had written several papers on the modeling of imperfections in MIMO transmitters and their impact on communication performance. I have been working on similar things but using far less sophisticated models.
The essence of the email was that he wanted us to write a paper together, but the circumstances came as a chock. Peter had been sick for a while and it turned out to be a terminal illness. He asked me to finalize a manuscript that he had initiated. I agreed and we exchanged a few emails but just as I and my postdoc were about to begin the editing of Peter’s manuscript, he passed away on September 15, 2019.
Impact of Backward Crosstalk in MIMO Transmitters
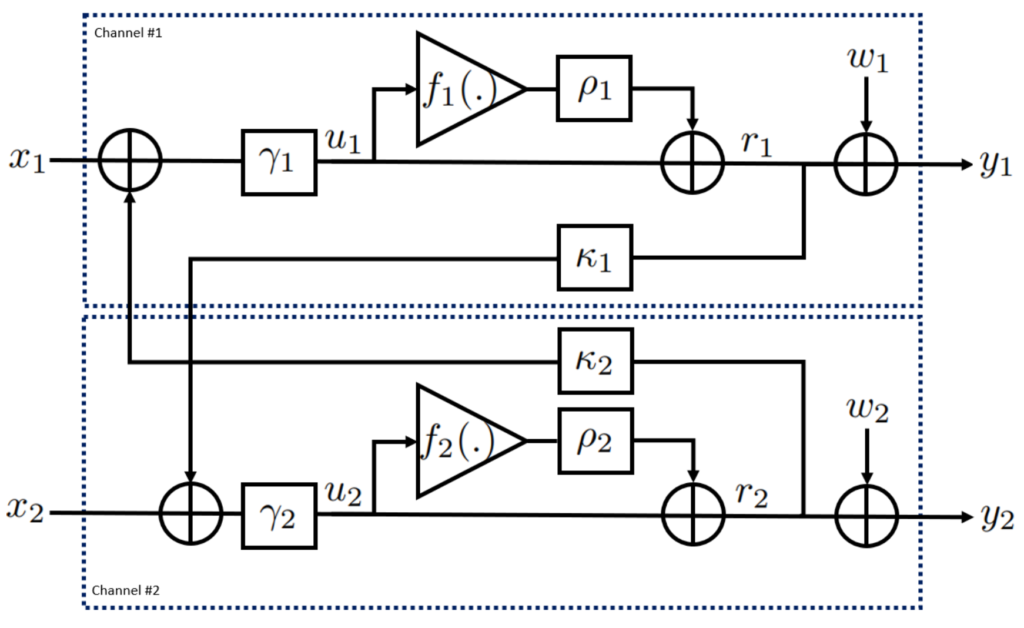
The manuscript considers a type of hardware impairment called backward crosstalk. It can be a major issue in the design of multi-antenna transmitters, but is generally overlooked by communication engineers. The issue arises when you build an antenna-integrated radio, for example, a Massive MIMO array with many antenna elements, power amplifiers, and radio-signal generators in a compact box. In this case, the output signal from one power amplifier will leak into the inputs of the neighboring power amplifiers. Even if the leakage is small in relative terms, it can still have a non-negligible impact since the output power of an amplifier is much higher than the input power. A small fraction of a large power value can still be rather large. In addition to this kind of backward crosstalk between amplifiers, there is also forward crosstalk in practice but it can be neglected for the very same reason.
We managed to finalize the manuscript, thanks to the excellent work by my postdoc Özlem Tuğfe Demir. The paper is now available:
Peter Händel, Özlem Tuğfe Demir, Emil Björnson, Daniel Rönnow, “Impact of Backward Crosstalk in 2×2 MIMO Transmitters on NMSE and Spectral Efficiency,” IEEE Transactions on Communications, vol. 68, no. 7, pp. 4277-4292, July 2020.
The paper considers a system model containing backward crosstalk, as well as, power amplifier non-linearities and transmitter noise. We characterize the performance both at the transmitter side (the normalized mean-squared error) and at the receiver side (the spectral efficiency). In turns out that optimization based on these two metrics can lead to very different transmission strategies; from a spectral efficiency perspective, one can transmit at higher power and accept a higher level of distortion since the desired signal power is also growing. In the paper, we also demonstrate how the precoding can be adapted to partially compensate for the crosstalk.
This paper is just a first step towards modeling real hardware imperfections that are normally ignored in academia or lumped together into a single additive term characterized by the error-vector magnitude. In the last emails I received from Peter, he expressed his view that there is a lot of open problems to solve at the interface between proper modeling of communication hardware and the design of signal processing schemes. I agree with him and encourage anyone who is looking for open problems on MIMO communications to have a closer look at his final papers on this topic:
- Impact of Backward Crosstalk in 2×2 MIMO Transmitters on NMSE and Spectral Efficiency
- MIMO and massive MIMO transmitter crosstalk
- Nonlinear distortion noise and linear attenuation in MIMO systems—Theory and application to multiband transmitters
- Modeling mixer and power amplifier impairments
- Dirty MIMO transmitters: Does it matter?
(I wrote this blog post in memoriam of Professor Peter Händel, who would have become 58 years today.)